List of typos:
P7,L5: First entry of G should be z_1 and second entry should be z_2.
P9, L2: \kappa(x) should be \kappa(z).
P27, Definition 2.3: The last sentence in the current definition
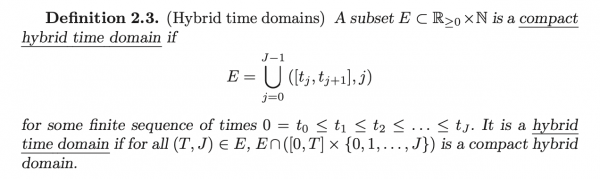

should be changed to
A set E \subset \realsgeq \times \nats is a hybrid time domain if it is the union of a nondecreasing sequence of compact hybrid time domains, namely, E is the union of compact hybrid time domains E_j with the property that E_0 \subset E_1 \subset E_2 \subset \ldots \subset E_j \ldots.
The issue with the current definition is as follows. Consider a set E to be the union of \realsgeq \times \{0\} and the point (0,1). Clearly, this is not the type of set we want to qualify as a hybrid time domain. Note that, for each (T,J) \in E, we do have that
E \cap ([0,T] \times \{0,1,\ldots,J\}
is a compact hybrid time domain. Indeed, either (T,J)=(T,0) or (T,J) = (0,1). In the former case, the intersection is [0,T] \times \{0\}. In the latter case, the intersection is (0,0) \cup (0,1). Both are clearly compact hybrid time domains.
P32, L4: \phi_d in the second formula should be \phi_d(1,j)=( (3/4)^j , 1/4 (3/4)^{j-1} )
P34, L-8: It is not the case that \phi_b jumps out of the union of the jump set and the closure of the flow set.
P37, L38: Equation t_{i_1+1}-t_{i_1}\geq \tau_D should be t_{i_k+1}-t_{i_k}\geq \tau_D.
P37, 11 lines below (2.5): "If \sigma in a solution" should be "If \sigma is a solution"
P38 Figure 2.8(a): caption should be “\tau_D smaller than or equal to...”
P38 L-3: f_q(\phi) should be f_q(z).
P39, L8: Since we are characterizing a solution to (2.5) which involves sigma and not q, it should say \sigma'(t)=q(t,j).
P53, L-8: the correct definition should be delta = alpha_2^{-1}( alpha_1 (epsilon) ), R = alpha_1^{-1} (alpha_2(r) ).
P54, L5: The subscript "{\cal A}" should be after the right vertical bar.
P60: the brackets are missing for the equation number in 'in place of bound 3.5'.
P62, L3 from beginning of proof: the sign of the exponent of e should be positive.
P101, first line of Definition 5.8: remove "Domain".
P102, sixth line of Definition 5.9: it should be "from R^m to R^n" instead of "from R^n to R^m".
P104, Example 5.13: the value of T_{S}(x) for x = a should be [0,\infty) and the value for x = b should be (-\infty,0].
P122: instead of "semicontinous mapping" it should be "semicontinuous mapping"
P129, 7th line of Example 6.20, it is not true that S_1 is weakly backward invariant and weakly invariant. The text right below S_1 should read "is weakly forward invariant (the forward invariance property is in fact "strong" as it holds for every solution to $\cal H$). The set $S_1$is not weakly backward invariant since points in the interior of the third and fourth quadrants cannot be reached for arbitrary large $\tau$."
P131, 4th line of proof of Prop. 6.26, "forward" should be "backward".
P136, the title of Proposition 6.35 (page 136) should be "non-Zenoness and non-continuousness".
P148: In Example 7.16, it should read:
"For the latter statement, note that for any function $\rho : \mathbb{R} \rightarrow \mathbb{R}_{\ge 0}$ with $\rho(1) > 0,$ …"
P148, in Lemma 7.17, it should A \subset U rather than A \subset BpA.
P161, in Example 7.35, first math display should have \nabla to the left of W(z). Same comment applies six lines before: \nabla should be added to the left of V(z).
List of clarifications:
The change to Definition 2.3 is due to the following. Consider a set E to be the union of \realsgeq \times \{0\} and the point (0,1). Clearly, this is not the type of set we want to qualify as a hybrid time domain. Note that, for each (T,J) \in E, we do have that
E \cap ([0,T] \times \{0,1,\ldots,J\}
is a compact hybrid time domain. Indeed, either (T,J)=(T,0) or (T,J) = (0,1). In the former case, the intersection is [0,T] \times \{0\}. In the latter case, the intersection is (0,0) \cup (0,1). Both are clearly compact hybrid time domains.
In Appendix: List of Symbols: Given sets A and B, the symbol \subset in "A \subset B" indicates that A is a subset of B, not necessarily a proper subset.
